To tylko jedna z 2 stron tej notatki. Zaloguj się aby zobaczyć ten dokument.
Zobacz
całą notatkę
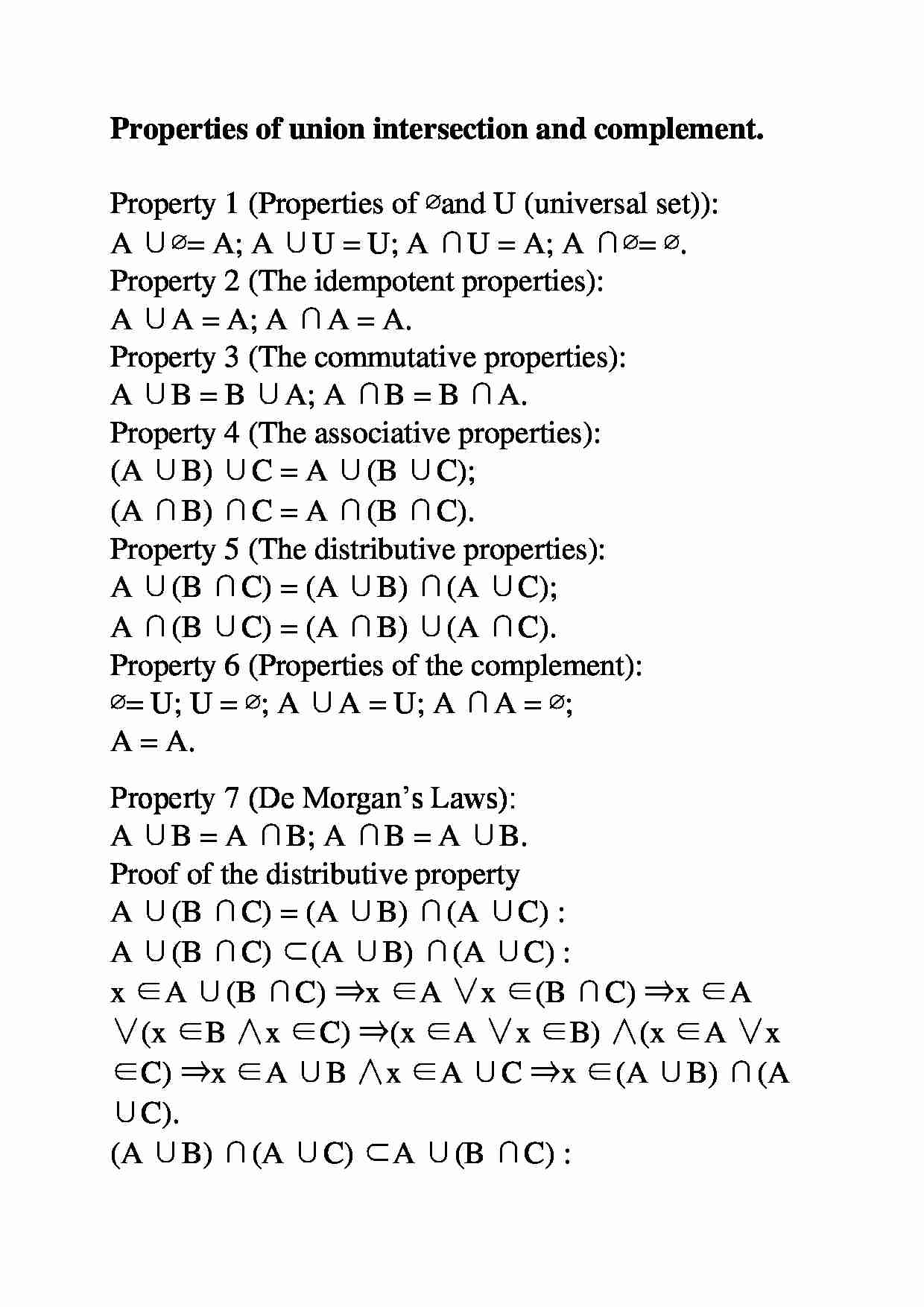

Properties of union intersection and complement. Property 1 (Properties of ∅ and U (universal set)): A ∪ ∅ = A ; A ∪ U = U ; A ∩ U = A ; A ∩ ∅ = ∅ . Property 2 (The idempotent properties): A ∪ A = A ; A ∩ A = A. Property 3 (The commutative properties): A ∪ B = B ∪ A ; A ∩ B = B ∩ A. Property 4 (The associative properties): ( A ∪ B ) ∪ C = A ∪ ( B ∪ C ); ( A ∩ B ) ∩ C = A ∩ ( B ∩ C ) . Property 5 (The distributive properties): A ∪ ( B ∩ C ) = ( A ∪ B ) ∩ ( A ∪ C ); A ∩ ( B ∪ C ) = ( A ∩ B ) ∪ ( A ∩ C ) . Property 6 (Properties of the complement): ∅ = U ; U = ∅ ; A ∪ A = U ; A ∩ A = ∅ ; A = A. Property 7 (De Morgan's Laws):
A ∪ B = A ∩ B; A ∩ B = A ∪ B.
Proof of the distributive property
A ∪ (B ∩ C) = (A ∪ B) ∩ (A ∪ C) :
A ∪ (B ∩ C) ⊂ (A ∪ B) ∩ (A ∪ C) :
x ∈ A ∪ (B ∩ C) ⇒ x ∈ A ∨ x ∈ (B ∩ C) ⇒ x ∈ A ∨ (x ∈ B ∧ x ∈ C) ⇒ (x ∈ A ∨ x ∈ B) ∧ (x ∈ A ∨ x ∈ C) ⇒ x ∈ A ∪ B ∧ x ∈ A ∪ C ⇒ x ∈ (A ∪ B) ∩ (A ∪ C).
(A ∪ B) ∩ (A ∪ C) ⊂ A ∪ (B ∩ C) :
x ∈ (A ∪ B) ∩ (A ∪ C) ⇒ x ∈ A ∪ B ∧ x ∈ A ∪ C ⇒ (x ∈ A ∨ x ∈ B) ∧ (x ∈ A ∨ x ∈ C) ⇒ x ∈ A ∨ (x ∈
B ∧ x ∈ C) ⇒ x ∈ A ∨ x ∈ (B ∩ C) ⇒ x ∈ A ∪ (B ∩ C).
... zobacz całą notatkę




Komentarze użytkowników (0)