To tylko jedna z 2 stron tej notatki. Zaloguj się aby zobaczyć ten dokument.
Zobacz
całą notatkę
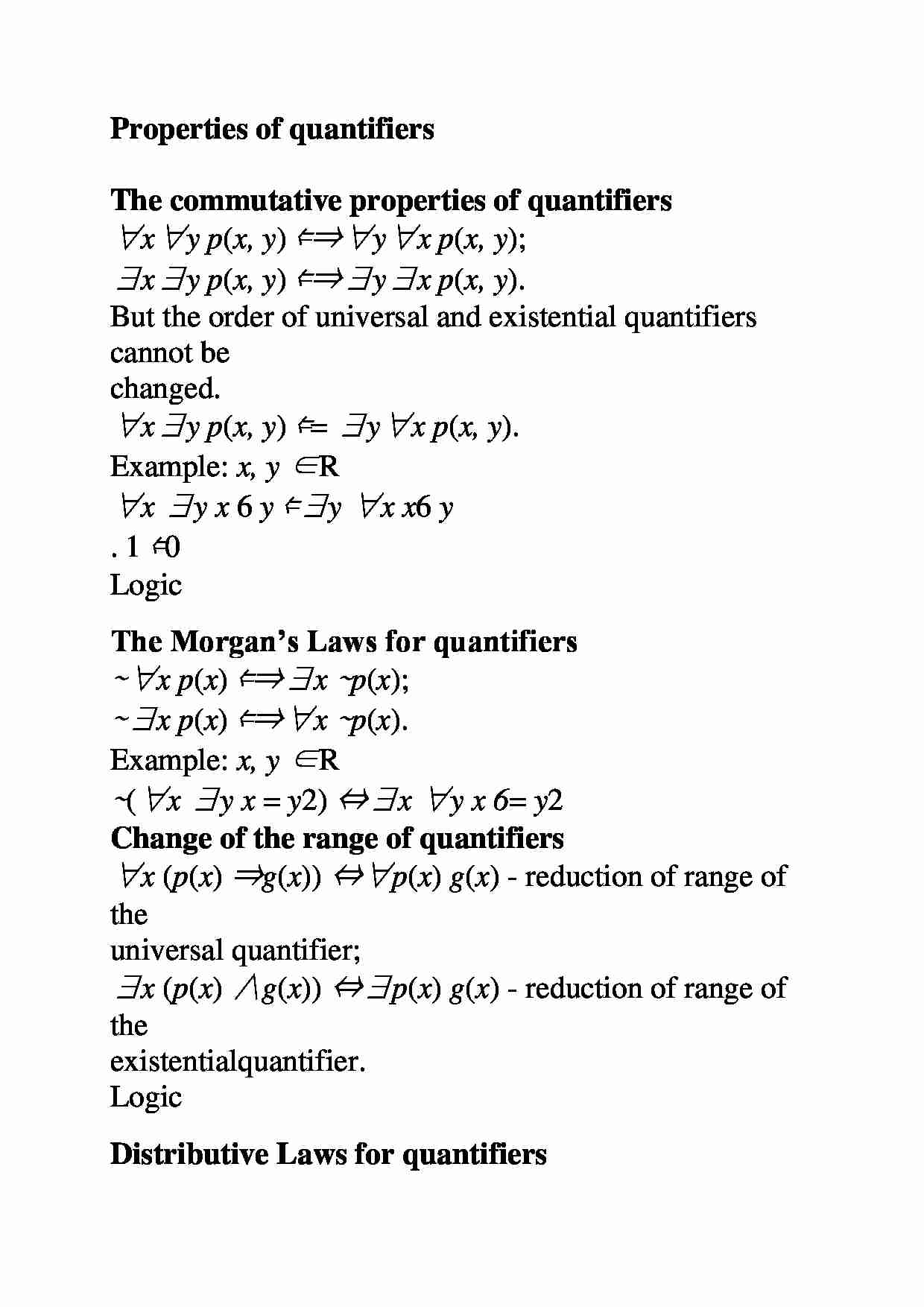

Properties of quantifiers The commutative properties of quantifiers ∀ x ∀ y p ( x, y ) ⇐⇒ ∀ y ∀ x p ( x, y ) ; ∃ x ∃ y p ( x, y ) ⇐⇒ ∃ y ∃ x p ( x, y ) . But the order of universal and existential quantifiers cannot be changed. ∀ x ∃ y p ( x, y ) ⇐ = ∃ y ∀ x p ( x, y ) . Example: x, y ∈ R ∀ x ∃ y x 6 y ⇐ ∃ y ∀ x x 6 y . 1 ⇐ 0 Logic The Morgan's Laws for quantifiers ∼ ∀ x p ( x ) ⇐⇒ ∃ x ∼ p ( x ) ; ∼ ∃ x p ( x ) ⇐⇒ ∀ x ∼ p ( x ) . Example: x, y ∈ R ∼ ( ∀ x ∃ y x = y 2 ) ⇔ ∃ x ∀ y x 6 = y 2 Change of the range of quantifiers ∀ x ( p ( x ) ⇒ g ( x )) ⇔ ∀ p ( x ) g ( x ) - reduction of range of the universal quantifier; ∃ x ( p ( x ) ∧ g ( x )) ⇔ ∃ p ( x ) g ( x ) - reduction of range of the existential quantifier. Logic Distributive Laws for quantifiers Distributive properties of universal quantifier ∀ x ( p ( x ) ∧ g ( x )) ⇔ ( ∀ x p ( x )) ∧ ( ∀ x g ( x )) . But: ∀ x ( p ( x ) ∨ g ( x )) ⇐ ( ∀ x p ( x )) ∨ ( ∀ x g ( x )) . Example: x ∈ R ∀ x ( x 6 0 ∨ x 0) ⇐ ( ∀ x x 6 0) ∨ ( ∀ x x 0) . 1 ⇐ 0 ∨ 0 Logic Distributive Laws for quantifiers Distributive properties of existential quantifier ∃ x ( p ( x ) ∨ g ( x )) ⇔ ( ∃ x p ( x )) ∨ ( ∃ x g ( x )) . But: ∃ x ( p ( x ) ∧ g ( x )) ⇒ ( ∃ x p ( x )) ∧ ( ∃ x g ( x )) . Example: x ∈ N ∃ x (2 | x ∧ ∼ (2 | x )) ⇒ ( ∃ x 2 | x ) ∧ ( ∃ x ∼ (2 | x )) . 0 ⇒ 1 ∧ 1 Logic
... zobacz całą notatkę




Komentarze użytkowników (0)