To tylko jedna z 3 stron tej notatki. Zaloguj się aby zobaczyć ten dokument.
Zobacz
całą notatkę
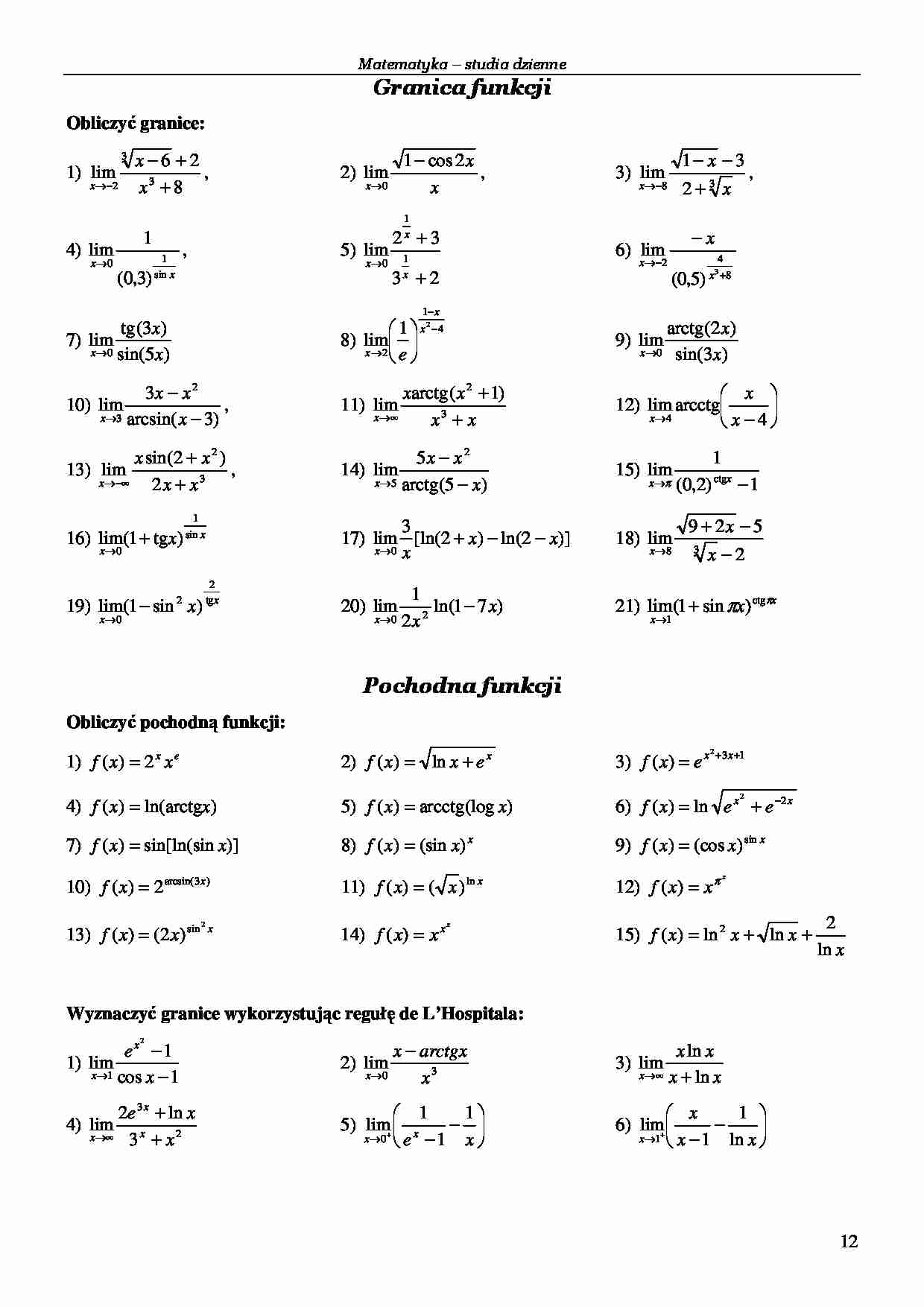


Matematyka – studia dzienne Granica funkcji Obliczyć granice: 3 x − 6 + 2 1 − cos 2 x 1 − x − 3 1) lim , 2) lim , 3) lim , 3 x → 2 − x + 8 x →0 x x → 8 − 3 2 + x 1 1 2 x + 3 − x 4) lim , 5) lim 6) lim x 1 →0 1 x →0 4 x → 2 − sin x ( ) 3 , 0 3 x + 2 3 x +8 ( ) 5 , 0 1− x tg 3 ( x ) 2 1 x −4 arctg(2 x ) 7) lim 8) lim 9) lim x →0 sin 5 ( x ) x →2 e x →0 sin 3 ( x ) 3 2 x − x x arctg( x 2 + ) 1 x 10) lim , 11) lim 12) lim arcctg x →3 arcsin( x − ) 3 x →∞ x 3 + x x →4 x − 4 2 x sin(2 + x ) 5 2 x − x 1 13) lim , 14) lim 15) lim 3 x →−∞ 2 x + x x →5 arctg 5 ( − x ) → ( , 0 2)ctg x x π −1 1 3 9 + 2 x − 5 16) x lim 1 ( + tg x sin ) 17) lim [ln 2 ( + x ) − ln(2 − x )] 18) lim x →0 x →0 x x 8 → 3 x − 2 2 1 19) 2 x lim 1 ( − sin x tg ) 20) lim ln 1 ( − 7 x ) 21) x lim 1 ( + sin x π π ctg ) x →0 x → 2 2 0 x x 1 → Pochodna funkcji Obliczyć pochodną funkcji: 2 1) x e f ( x ) = 2 x 2) x f ( x ) = ln x + e 3) x +3 1 f ( x ) + = x e 2 4) f ( x ) = ln a ( rctg x ) 5) f ( x ) = arcctg(log x ) 6) x −2 x f ( x ) = ln e + e 7) f ( x ) = sin[ln(sin x )] 8) x f ( x ) = (sin x ) 9) sin x f ( x ) = (cos x ) x 10) arcsin(3 x ) f ( x ) = 2 11) ln x f ( x ) = ( x ) 12) f ( x ) x π = 2 x 2 2 13) sin x f ( x ) = (2 x ) 14) x f ( x ) = x 15) f ( x ) = ln x + ln x + ln x Wyznaczyć granice wykorzystując regułę de L’Hospitala: 2 e x −1 x − arctgx x ln x 1) lim 2) lim 3) lim x 1 → cos x −1 3 x →0 x x →∞ x + ln x 3 2 e x + ln x 1 1 x 1 4) lim 5) lim − 6) lim − x 2 x →∞ 3 + x + → + 0 e x x −1 x x →1 x − 1 ln x 12 Matematyka – studia dzienne 1 7) lim tg x ⋅ ln(sin x ) 8) lim ln x ⋅ ln 1 ( − x ) 9) x lim x e −1 −
(…)
… przegięcia, przedziały wklęsłości i wypukłości
Określić przedziały wklęsłości i wypukłości oraz wyznaczyć punkty przegięcia wykresu funkcji:
1) f ( x) =
1 2
x + ln( x + 1)
2
4) f ( x) = xe
1
x
2) f ( x) = x 2 ⋅ e − x
5) f ( x) = xe
x2
3) f ( x) = x ⋅ ln(4 x − 1)
6) f ( x) = e
−2
x
1
x
7) f ( x) = x 4 − x 2
8) f ( x) = 3 x − 1 + x
9) f ( x) = arcctg
10) f ( x) = ln 2 x − 2 ln x
11) f ( x) = 3 ( x − 1) 2
12) f ( x) = x ln(4 − x 2 )
13) f ( x) = x ln x − x 2
14) f ( x) = arcctgx + ln(1 + x 2 )
15) f ( x) = (ln x − 2) ln x
16) f ( x) = e arctgx
17) f ( x) = e x − ex
1
18) f ( x) = x ln e +
x
2) f ( x) = e − x
3) f ( x) =
1
Narysować wykres funkcji:
1
1) f ( x) = x 2 e x
4) f ( x) =
x
ln x
2
5) f ( x) = x + 3arctgx
1
e −1
x
2x
6) f ( x) = arcsin
2
1+ x
14
…
… →1
(
x→0
)
)
tgx
12) lim− ( tgx) cos x
x→
2
14) lim arctgx
x→∞ π
ln x
16) lim e 2 x + x
(
11) lim sin 2 x
+
10) lim
x
1
1
x
π
2
1
15) lim
+
x →0 x
tgx
18) lim (tgx ) ln x
+
1
17) lim+ (cos x) x
19) lim ( x) sin
+
x →0
x→0
2
x
x →0
Asymptoty wykresu funkcji
Wyznaczyć asymptoty wykresu funkcji:
1) f ( x) =
x4
8 − x3
2) f ( x) =
x3
( x − 2) 2
3) f ( x) =
x2
2− x
1
4) f ( x) = 2 x + arctgx
1
5) f ( x) = e 1− x
6) f ( x) = e x − x
1
x
x
7) f ( x) =
ln x
8) f ( x) = xe
10) f ( x) = x 2 ln x
11) f ( x) = ln(4 − x 2 )
1
13) f ( x) = x ⋅ ln
x
14) f ( x) = ln x +
x
16) f ( x) = 3 x − 2arcctg
3
17*) f ( x) = x x
9) f ( x) =
1
ln x
1
e −1
x
12) f ( x) = x ⋅ arctgx
15) f ( x) = arctgx + x 2 + 2
18*) f ( x) = x sin x
* w przykładach 17, 18 wyznaczyć tylko asymptoty pionowe…
…
Ekstrema lokalne funkcji jednej zmiennej
Określić przedziały monotoniczności i wyznaczyć ekstrema lokalne funkcji:
1 2 x2
e
x
1) f ( x) = x 3 e − x
2) f ( x) =
ln 2 x
4) f ( x) =
x
5) f ( x) = e x − 1
6) f ( x) = arcsin
8) f ( x) = ln(2 x + 1) − x 2
9) f ( x) = 2 x − 1 ⋅ e1− 2 x
11) f ( x) = ln 3 x − 3 ln x
12) f ( x) =
7) f ( x) = ( x − 2)e
10) f ( x) =
2
1
x −2
1
+ ln(2 x − 1)
2x − 1
3) f ( x) = x 3…
... zobacz całą notatkę






Komentarze użytkowników (0)