To tylko jedna z 2 stron tej notatki. Zaloguj się aby zobaczyć ten dokument.
Zobacz
całą notatkę
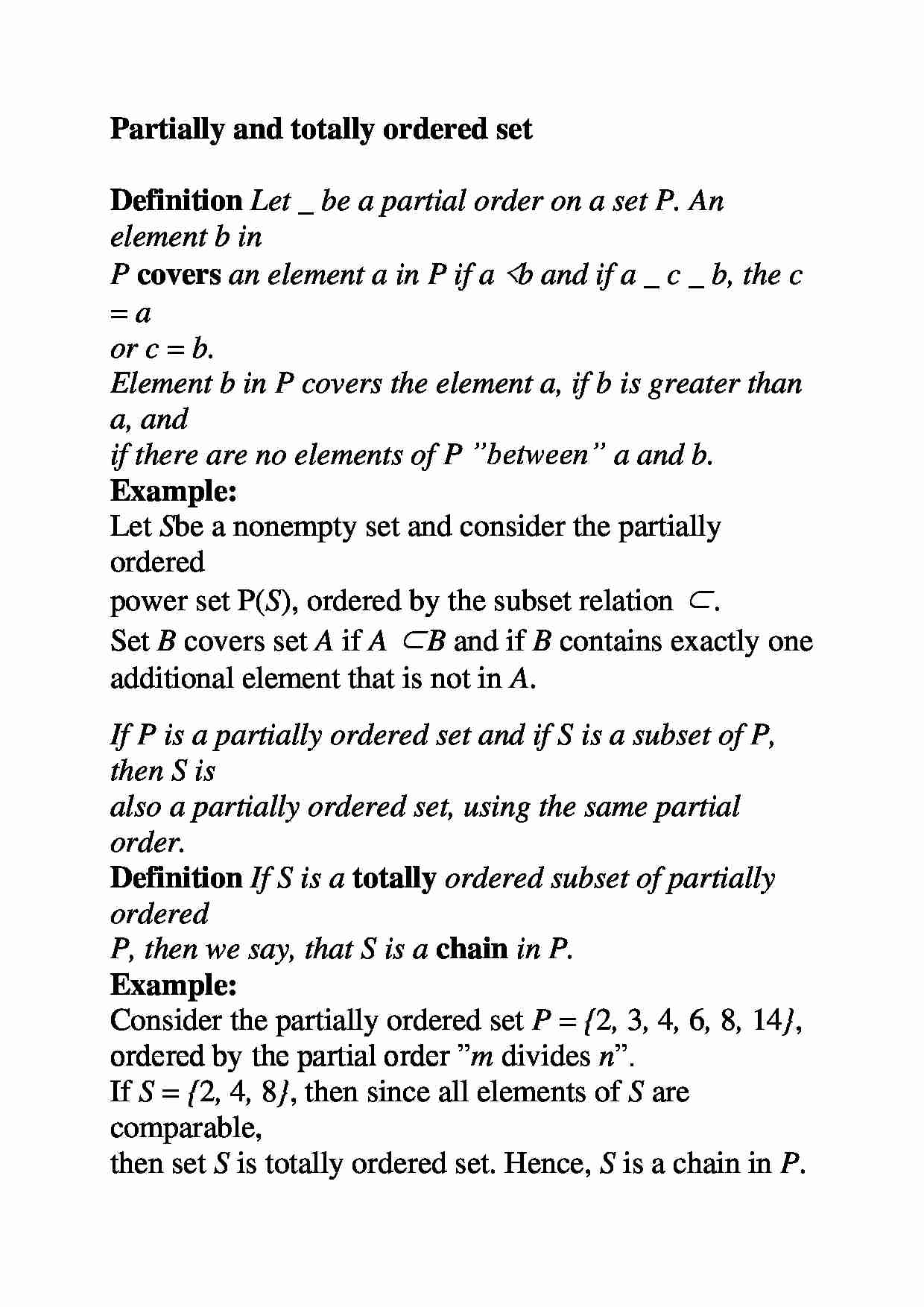

Partially and totally ordered set Definition Let _ be a partial ord er on a set P . An element b in P covers an element a in P if a ≺ b and if a _ c _ b , the c = a or c = b . Element b in P covers the element a , if b is greater than a , and if there are no elements of P ”between” a and b . Example: Let S be a nonempty set and consider the partially ordered power set P ( S ) , ordered by the subset relation ⊂ . Set B covers set A if A ⊂ B and if B contains exactly one additional element that is not in A . If P is a partially ordered set and if S is a subset of P , then S is also a partially ordered set, using the same partial order. Definition If S is a totally ordered subset of partially ordered P , then we say, that S is a chain in P . Example: Consider the partially ordered set P = { 2 , 3 , 4 , 6 , 8 , 14 } , ordered by the partial order ” m divides n ”. If S = { 2 , 4 , 8 } , then since all elements of S are comparable, then set S is totally ordered set. Hence, S is a chain in P . Relations on
... zobacz całą notatkę




Komentarze użytkowników (0)